I posted a picture on Twitter last week about my curriculum for my last Games and Game Theory class. I guess folks were surprised that it was really just a big stack of games? I’m sure that I have a syllabus somewhere but, like my students, I probably recycled it as soon as the first class was over. The whole point of the class is to find out how game theory shows up in the board games we all know and love, and these games exemplify some of those important problems. Today I want to talk about two of them in particular and how they relate to the terrible study of probability.
Mathematicians like to say that if you think you understand probability, you’re probably wrong. Actually, that might just be me, but it is certainly true that probability is notoriously counter-intuitive. Sure, a coin can only flip heads or tails. Most people, when shown a six-sided die, will come to the conclusion that a 2 is just as likely of a result as a 5. But a far fewer number will be able to explain why on a pair of six-sided dice, a 2 is absolutely not as likely a result as a 5. Even fewer are equipped to easily explain why in exact terms. Did you know that any random group of 23 people has a 50% chance that two group members will have the same birthday? Doesn’t that number seem ridiculously small? And all of this is long before we get to ideas about probability distributions and confidence intervals.
Okay fine. Let’s stop for a moment and answer a few of your questions.
1. No, a coin cannot land on its edge. If it magically did, we wouldn’t count it anyway. In a heads-or-tails game, it’s a redo.
2. Sure, dice are made in ways that make them inherently unfair, but these are generally ignorable, depending on the rock crusher.
3. While the results of two six-sided dice seem like a a list of 12 outcomes, there are really 36. Et voilà!
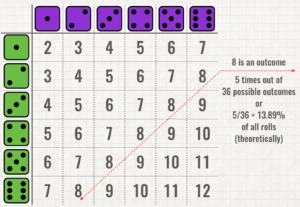
Games that are based on two dice make these fundamental properties of probabilities more intuitive. Machi Koro and Valeria: Card Kingdoms are two incredible tableau-building games, where you purchase cards which will earn currencies when certain results are rolled on the dice each round. At the start, you only earn on a few numbers, but as you purchase more, you start to reap a serious harvest.
In Machi Koro, you are trying to build a city filled with businesses and attractions. On your turn, you roll and collect gold for every business you have showing the same number at the top. As you progress through the game, you have the option of rolling one or two dice. The businesses that trigger on 7 through 12 may be a little more glamorous, but those low numbers can keep you chugging along through the game for quite some time. At some point, every player has to make the switch, and the game suddenly changes dramatically. Machi Koro also has restaurants, which allow you to steal gold from players when certain numbers come up, and that makes this game feel pretty mean and hopeless sometimes. In the end, players need to use probability, luck, and an efficient engine to build their attractions before anyone else.
While similar in style, Valeria: Card Kingdoms has some great tweaks that makes it a very fun and engaging game. With very little inter-player conflict, everyone is free to hoard resources all they want, and they come quickly! Every card has a benefit you gain either when you roll its number, or when another player rolls it on their turn. Valeria ramps up like no game I’ve ever seen. While early turns may net you just a single gold, by the end of the game, one lucky roll of the dice can easily net you twenty or more currency. With multiple paths to victory, players are free to choose exactly what kind of citizens they want in their city, monsters they want to destroy, and domains to add to their kingdoms. This game is always a contender at my game nights!
My poor, beleaguered students had to play these two games and study the results of their choices. Would they do better in these games if they focused on a few numbers with maximum results, or would they take the broad approach and try to earn on every single possible roll of the dice? Playing around with these play styles is an excellent way for anyone to try to make the arcane study of probabilities infinitely more understandable and intuitive.
4. Are you still thinking about the birthday problem? Here’s the trick. I never said it was someone else who has the same birthday as YOU. That’s a whole different story.
UPDATE: Daily Magic Games challenged me to create a chart listing the exact probabilities for each outcome in Valeria: Card Kingdoms! So, of course…
